Wannier函數是評估固體材料中各種電子性質的有力工具,其應用范圍涵蓋電極化和軌道磁化以及拓撲性質等。此外,Wannier函數提供了一種通過緊湊的漢密爾頓方法,用以描述晶體中的能帶,因此可以在不需要額外調用代碼的情況下,快速評估布里淵區中任何一點的能帶結構。
這種Wannier插值方法在原理上類似于緊束縛方法,但具有顯著的優勢。Wannier插值提供了一個系統方法,在不截斷跳躍積分的情況下,可精確描述任何數量的能帶,相比之下,傳統的緊束縛方法因基組數量有限而不可避免地出現誤差。此外,該方法還可以精確地插值能帶的能量、各種觀測值和算符的矩陣元。
這類插值在尋找能帶結構中的外爾點、評估快速變化函數的動量空間積分時,都特別有用。這類積分包括反常霍爾電導、軌道磁化、玻耳茲曼輸運系數和光學性質的計算等。
目前,已有多種Wannier插值方案,已用于需在布里淵區進行密集采樣的性質計算中,如電子-聲子耦合、陀螺效應和自旋霍爾電導等。然而,更復雜的物理問題和材料帶來了更難的數值計算挑戰,用現有的代碼計算將變得非常昂貴,阻礙Wannier插值方法所能達到的預期精度。
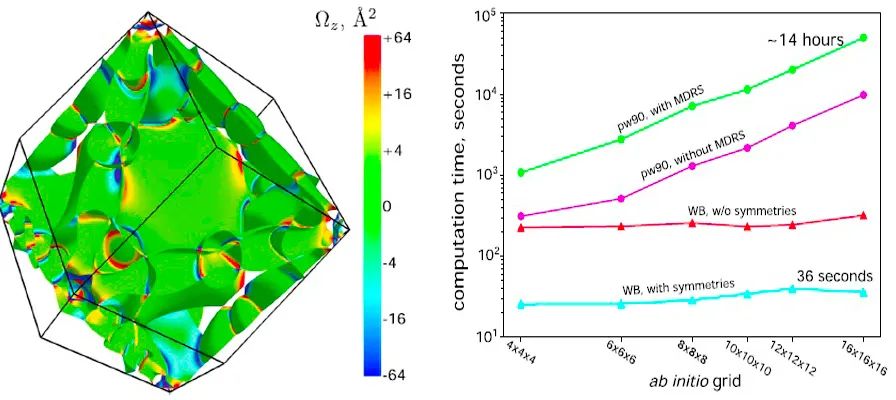
來自瑞士蘇黎世大學物理系的Stepan S. Tsirkin教授提出了一系列方法,將Wannier插值的性能提升到了更高的水平。這些方法是在Python代碼WannierBerri中實現的。混合并優化了傅里葉變換和最小距離復制選擇法(MDRS),Fermi能級迭代不僅在計算上有很大優勢,而且并不影響計算精度。
因此,WannierBerri可以很容易地用已建立的postw90.x代碼進行基準測試。該代碼不僅可以對反常霍爾電導率和其他屬性作高速度和高精度的計算,還可以作為平臺來實現更多Wannier插值的功能。因此,它有可能成為一個社區代碼。有趣的是,該代碼使用同一程序來執行基于Wannier函數和緊束縛模型的計算。
該文近期發表于npj Computational Materials 7: 33 (2021),英文標題與摘要如下。

High performance Wannier interpolation of Berry curvature and related quantities with Wannier Berri code
Stepan S. Tsirkin
Wannier interpolation is a powerful tool for performing Brillouin zone integrals over dense grids of k points, which are essential to evaluate such quantities as the intrinsic anomalous Hall conductivity or Boltzmann transport coefficients.
However, more complex physical problems and materials create harder numerical challenges, and computations with the existing codes become very expensive, which often prevents reaching the desired accuracy. In this article, I present a series of methods that boost the speed of Wannier interpolation by several orders of magnitude.
They include a combination of fast and slow Fourier transforms, explicit use of symmetries, and recursive adaptive grid refinement among others. The proposed methodology has been implemented in the python code WannierBerri, which also aims to serve as a convenient platform for the future development of interpolation schemes for other phenomena.
編輯:jq
-
電子
+關注
關注
32文章
1898瀏覽量
89546 -
函數
+關注
關注
3文章
4345瀏覽量
62884 -
代碼
+關注
關注
30文章
4823瀏覽量
68904 -
python
+關注
關注
56文章
4807瀏覽量
84961
原文標題:npj: 更精確電子結構的展示—新軟件的作用
文章出處:【微信號:zhishexueshuquan,微信公眾號:知社學術圈】歡迎添加關注!文章轉載請注明出處。
發布評論請先 登錄
相關推薦
示波器波形分析軟件使用指南
滑動變阻器的作用是什么?

柔性電子技術革新與人形機器人的電子皮膚應用前景




 更精確電子結構的展示—新軟件的作用
更精確電子結構的展示—新軟件的作用












評論